Not a wrinkle in time: many orderly dimensions of spacetime
“Wanna see something cool?” is a question I ask quite frequently at mass social engagements now. It’s usually queried unsolicited of some hapless chap who made the mistake of letting the conversation dwindle to an awkward silence.

Yes. This is how I interact with people.
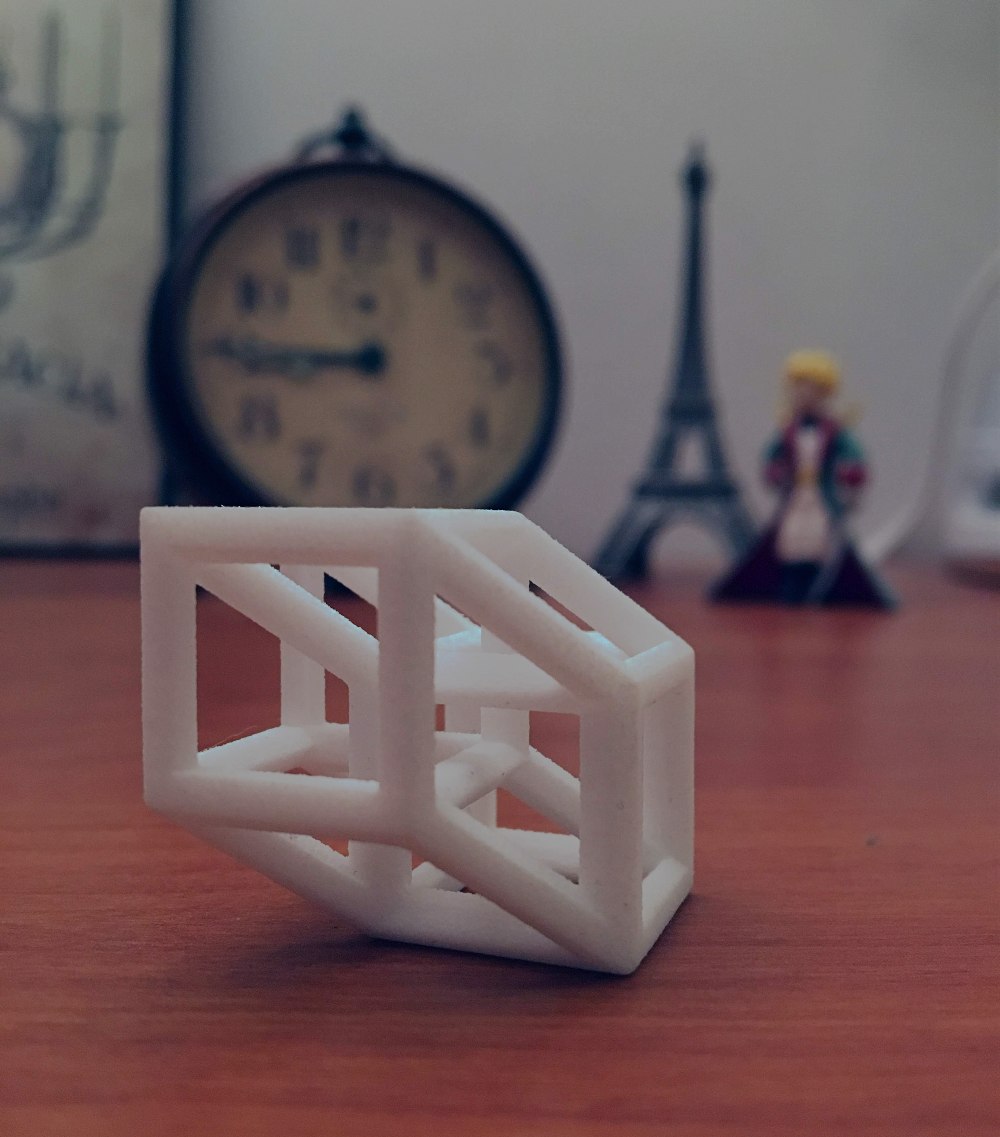
But the question is unnecessary and redundant for two reasons; 1) who doesn’t want to see something cool? and 2) before they have the time to answer, I’ve unsheathed from my pocket a small, white silicon structure reminiscent of a large-scale molecule from Chemistry 101.
“What’s that?” they ask.
“It’s a tesseract,” I say, grinning because I’ve effectively manipulated them into a twenty-minute one-sided discussion about dimensions and space and time and spacetime.
Look! Spacetime fits in my mouth!
Yes. This is how I interact with people. More about my tomfoolery here.
So what’s a tesseract? It’s not a wrinkle in time, as Madeleine L’Engle claimed in her famous fiction series.
Mathematically speaking, a tesseract is a cube in four-dimensions. The object my partner gave me for Christmas last year is a three-dimensional representation of that four-dimensional cube.
This is quite a lot to wrap one’s head around, so let’s start with conceiving of four-dimensional objects.
A zero-dimensional object is a point.To get from zero dimensions to one dimension, you take two zero-dimensional objects and connect them with a line. This gives you one line; a one-dimensional object.
We employ the same algorithm to get from one dimension to two: take two lines and connect the vertices with lines. This gives you a square. Then take this square and duplicate it, connect all the vertices and voila! A cube appears.
You’ve probably got a sense of the pattern now. So, to get from three dimensions to four dimensions, we take two cubes and connect their vertices with lines. Lo and behold, a tesseract.The dimensionality doesn’t stop there. In fact, string theory predicts our universe is permeated by 11 dimensions: 10 dimensions of space and one of time. Generations of hypercubes are defined precisely via this algorithm of duplicating and connecting dimensional objects to generate those of higher dimensions. I’ve neatly organized these for you in the following table.
| Dimension | Name |
| 0 | Monon (point) |
| 1 | Line |
| 2 | Square |
| 3 | Cube |
| 4 | Tesseract |
| 5 | Penteract |
| 6 | Hexeract |
| 7 | Hepteract |
| 8 | Octeract |
| 9 | Enneract |
| 10 | Dekeract |
Of course, we as humans and the objects we create are incarcerated by three dimensions; that’s why my pocket-size tesseract has lines that are not all orthogonal—or 90 degrees—to one another. This effect is similar to the way one draws a cube on a whiteboard; some of the angles must compromise due to the dimensional limitation. However, if time were a spatial dimension which we could perceive, we would see the tesseract as an object with all 90-degree angles as we do the cube. In this way, the tesseract is an object that represents spacetime. (See Hereby the Maverick post We are such stuff that spacetime is made of).
“And look! Spacetime fits in my mouth!”
My brain just shifted into a different gear. Thank you